What is Factor of Safety (FOS) in Civil Engineering Design?

The factor of safety (FOS) measures how much times greater the load-carrying capacity of a structural component is, than what is required. It is defined as the ratio of the capacity of the material to the demand for the material. 

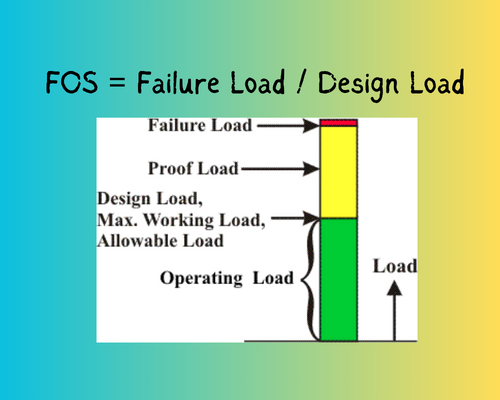Every structure and safety equipment in the construction and infrastructure industry is designed by considering the factor of safety. We determine the required overload expected for each unit to remain safe during an emergency. Hence, for more reliability, structures are built stronger than necessary. This way engineers can ensure that the structures are capable of withstanding greater loads than what they are expected to experience during their service life.

A structural component for example a beam needs to be designed to take up a maximum expected load of 100 tonnes. But we designed the beam for taking up to 250 tonnes. This extra increase of design load by 2.5 is to consider the risk of failure of the structure and overall safety. If we design the beam only for 100 tonnes, any unexpected increase in load would result in the collapse of the structure. 

In general, the factor of safety (FOS) can be expressed as:

Factor of Safety (FOS) = Allowable Stress or Ultimate Load Capacity / Actual Stress/Expected Load

or

Factor of Safety (FOS) = Actual Capacity/Demand Capacity

It must be noted that F.O.S. will always be greater than 1. This means the capacity of a material must always be greater than the demand on that material, in other words, the strength of a given material must be greater than the stress expected on the material.

Based on the above example:

Then, F.O.S can be calculated as:
F.O.S = strength of the beam/stress on the beam = 250/100 = 2.5.

Factor of Safety for Ductile and Brittle Materials

FOS value is used in the design of different structural members based on their behavior during their failure. In general,

FOS of any material = Maximum Stress / Design Stress

Brittle Materials

In the case of brittle materials, the failure would be sudden during unexpected loads. Here we use the ultimate strength as maximum stress to calculate the safety factor of brittle materials. 

FOS for Brittle Material = Ultimate Stress/ Design Stress = σut / σ design

Brittle failure occurs immediately after the elastic limit with small deformation. Hence, fracture and ultimate stress are the same in brittle materials, while calculating FOS.

Brittle materials have a non-homogeneous structure with residual stresses in the component. So for safety, a large factor of safety is used. Providing a higher value of F.O.S. during the design to provide enough room for evaluation or evacuation. The ultimate strength determines the safety factor until the failure.

Stress Strain Curve for Brittle and Ductile Materials

Ductile Materials

For ductile material, the maximum stress used is yield strength to determine the safety factor. 

FOS for Ductile Material = Yield Stress/ Design Stress = σyt / σ design

Beyond the yield strength of the material, the material is subjected to plastic deformation. Yield strength determines the factor of safety until the start of the deformation. But this state does not put the component to failure. 

Hence, we provide a low value of F.O.S to the design of these structural members. For instance, structures like slabs and beams have ductile failure. They reveal their path to failure through hairline cracks before sudden collapse, leaving time for evaluation or evacuation. 

If the structure is more Ductile, Low F.O.S and if more Brittle, High F.O.S.

So, in a graphical sense, you can think of the factor of safety as the vertical distance between the applied stress and the allowable stress on the stress-strain curve. The larger this distance, the safer the structure is from failure. It represents the margin of safety designed into the structure to account for uncertainties and potential variations in loading and material properties.

Factor of Safety in WSM and LSM Design Method

In the Working Stress Method (WSM), the safety margin (FOS) is usually set higher because it assumes worst-case scenarios for loads and materials, aiming for a very safe design.

On the other hand, the Limit State Method (LSM) uses a more modern approach that considers uncertainties more precisely. It uses different safety factors for different situations, allowing for a safer yet more efficient design with lower safety margins.

This shift to LSM is based on advanced engineering practices and a better understanding of materials, making it possible to create safe structures with lower safety margins, which can be more cost-effective.

Read More: Working Stress Method (WSM) vs Limit State Method (LSM) in Structural Engineering